Review:
Toward Comparative Collective Behavior to Discover Fundamental Mechanisms Underlying Behavior in Human Crowds and Nonhuman Animal Groups
Hisashi Murakami*1,†
 , Masato S. Abe*2,*3,*4
, Masato S. Abe*2,*3,*4
 , and Yuta Nishiyama*5
, and Yuta Nishiyama*5
*1Faculty of Information and Human Science, Kyoto Institute of Technology
Matsugasakihashigami-cho, Sakyo-ku, Kyoto, Kyoto 606-8585, Japan
†Corresponding author
*2Faculty of Culture and Information Science, Doshisha University
1-3 Tatara Miyakodani, Kyotanabe, Kyoto 610-0394, Japan
*3Advanced Intelligence Project, RIKEN
1-4-1 Nihonbashi, Chuo-ku, Tokyo 103-0027, Japan
*4Center for Brain Science, RIKEN
2-1 Hirosawa, Wako, Saitama 351-0198, Japa
*5Information and Management Systems Engineering, Nagaoka University of Technology
1603-1 Kamitomiokamachi, Nagaoka, Niigata 940-2188, Japan
This article provides comparative perspectives on collective behaviors that are widely found throughout the animal kingdom, ranging from insect and crustacea swarms, fish schools, bird flocks, and mammal herds to human crowds. Studies of nonhuman animal and human collective behaviors have progressed almost separately even though they have a similar history. Theoretical studies have investigated the reproduction of collective phenomena from simple inter-individual rules, and subsequent empirical and experimental studies have found diverse and complex collective behaviors that are difficult to explain with classical theoretical models. As a consequence, a wide variety of interaction rules have been proposed. To determine models to be implemented in nature and find fundamental mechanisms of collective behaviors, this paper argues that we should compare collective behaviors among various species while adopting Tinbergen’s four questions regarding mechanism, function, development, and evolution as a methodological basis. As an example of a comparative collective behavior paradigm, we introduce our studies in which a mutual anticipation mechanism inspired by nonhuman animal collective behaviors can be linked to a self-organization function in human collective behaviors. We expect that the study of comparative collective behaviors will expand, the methodology will become more sophisticated, and new perspectives regarding the multitemporal features of collective behaviors will emerge.
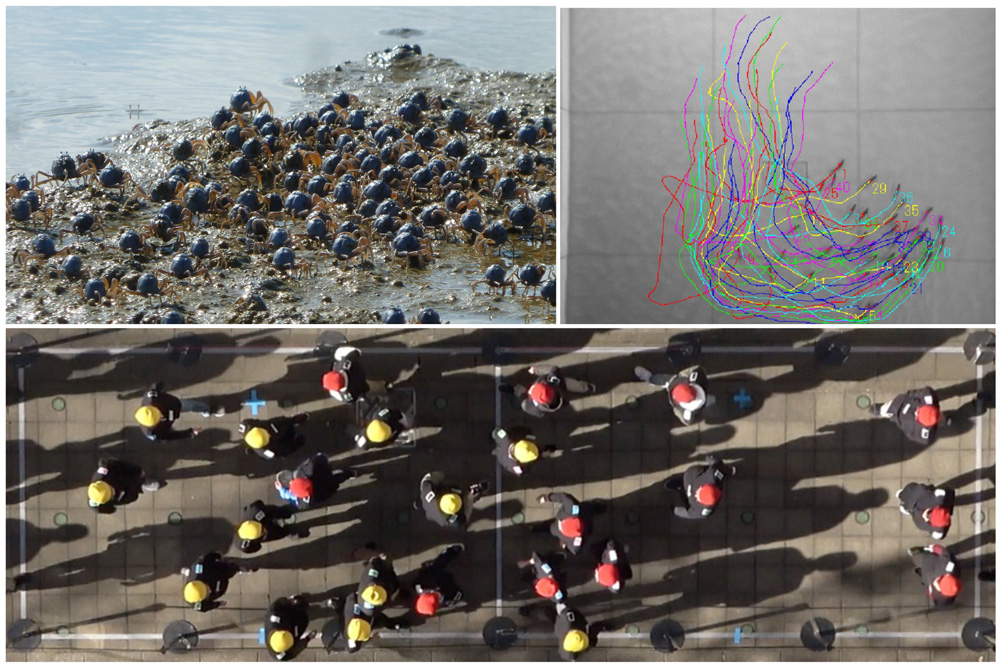
Toward comparative collective behavior
- [1] I. D. Couzin, “Collective minds,” Nature, Vol.445, p. 715, 2007. https://doi.org/10.1038/445715a
- [2] J. K. Parrish and L. Edelstein-Keshet, “Complexity, pattern, and evolutionary trade-offs in animal aggregation,” Science, Vol.284, pp. 99-101, 1999. https://doi.org/10.1126/science.284.5411.99
- [3] D. Sumpter, “Collective animal behavior,” Princeton University Press, 2010.
- [4] I. D. Couzin, “Collective cognition in animal groups,” Trends in Cognitive Sciences, Vol.13, No.11, pp. 517-524, 2009. https://doi.org/10.1016/j.tics.2008.10.002
- [5] C. W. Reynolds, “Flocks, Herds, and Schools: A Distributed Behavioral Model,” Comput. Graph., Vol.21, No.4, pp. 25-34, 1987. https://doi.org/10.1145/37402.37406
- [6] I. Aoki, “A Simulation Study on the Schooling Mechanism in Fish,” Bull. Jpn. Soc. Fish., Vol.40, pp. 1081-1088, 1982. https://doi.org/10.2331/suisan.48.1081
- [7] A. Okubo, “Dynamical aspects of animal grouping: swarms, schools, flocks, and herds,” Adv. Biophys., Vol.22, pp. 1-94, 1986. https://doi.org/10.1016/0065-227X(86)90003-1
- [8] C. K. Hemelrijk, H. Hildenbrandt, J. Reinders, and E. J. Stamhuis, “Emergence of oblong school shape: models and empirical data of fish,” Ethology, Vol.116, No.11, pp. 1099-1112, 2010. https://doi.org/10.1111/j.1439-0310.2010.01818.x
- [9] I. D. Couzin, J. Krause, R. James, G. D. Ruxton, and N. R. Franks, “Collective memory and spatial sorting in animal groups,” J. Theor. Biol., Vol.218, No.1, pp. 1-11, 2002. https://doi.org/10.1006/jtbi.2002.3065
- [10] I. L. Bajec, N. Zimic, and M. Mraz, “Simulating flocks on the wing: the fuzzy approach,” J. Theor. Biol., Vol.233, No.2, pp. 199-220, 2005. https://doi.org/10.1016/j.jtbi.2004.10.003
- [11] Y. Vicsek, A. Czirok, E. Ben-Jacob, and O. Shochet, “Novel Type of Phase Transition in a System of Self-Driven Particles,” Phys. Rev. Lett., Vol.75, No.6, pp. 1226-1229, 1995. https://doi.org/10.1103/PhysRevLett.75.1226
- [12] J. Buhl et al., “From Disorder to Order in marching locusts,” Science, Vol.312, No.5778, pp. 1402-1406, 2006. https://doi.org/10.1126/science.1125142
- [13] J. Toner and S. Ramaswamy, “Hydrodynamics and phases of flocks,” Ann. Phys., Vol.318, No.1, pp. 170-244, 2005. https://doi.org/10.1016/j.aop.2005.04.011
- [14] J. Toner and Y. Tu, “Long-range order in a two-dimensional dynamical XY model: how birds fly together?,” Phys. Rev. Lett., Vol.75, pp. 4326-4329, 1995. https://doi.org/10.1103/PhysRevLett.75.4326
- [15] J. Toner and Y. Tu, “Flocks, herds, and schools: a quantitative theory of flocking,” Phys. Rev. E, Vol.58, pp. 4828-4858, 1998. https://doi.org/10.1103/PhysRevE.58.4828
- [16] Y. Tu, J. Toner, and M. Ulm, “Sound waves and the absence of Galilean invariance in flocks,” Phys. Rev. Lett., Vol.80, pp. 4819-4822, 1998. https://doi.org/10.1103/PhysRevLett.80.4819
- [17] D. J. G. Pearce, A. M. Miller, G. Rowlands, and M. S. Turner, “Role of projection in the control of bird flocks,” Proc. Natl Acad. of Sci. USA, Vol.111, No.29, pp. 10422-10426, 2014. https://doi.org/10.1073/pnas.1402202111
- [18] M. Ballerini et al., “Empirical investigation of starling flocks: A benchmark study in collective animal behavior,” Anim. Behav., Vol.76, pp. 201-215, 2008. https://doi.org/10.1016/j.anbehav.2008.02.004
- [19] M. Ballerini et al., “Interaction ruling animal collective behavior depends on topological rather than metric distance: evidence from a field study,” Proc. Natl Acad. Sci. USA, Vol.105, No.4, pp. 1232-1237, 2008. https://doi.org/10.1073/pnas.0711437105
- [20] Y. Katz, K. Tunstrøm, C. C. Ioannou, C. Huepe, and I. D. Couzin, “Inferring the structure and dynamics of interactions in schooling fish,” Proc. Natl Acad. Sci. USA, Vol.108, No.46, pp. 18720-18725, 2011. https://doi.org/10.1073/pnas.1107583108
- [21] A. Cavagna, S. M. D. Queirós, I. Giardina, F. Stefanini, and M. Viale, “Diffusion of individual birds in starling flocks,” Proc. R. Soc. B, Vol.280, pp. 1471-2954, 2013. https://doi.org/10.1098/rspb.2012.2484
- [22] S. Bazazi, F. Bartumeus, J. J. Hale, and I. D. Couzin, “Intermittent Motion in Desert Locusts: Behavioral Complexity in Simple Environments,” PLoS Comput. Biol., Vol.8, Article No.e1002498, 2012. https://doi.org/10.1371/journal.pcbi.1002498
- [23] J. Buhl et al., “From Disorder to Order in Marching Locusts,” Science, Vol.312, No.5778, pp. 1402-1406, 2006. https://doi.org/10.1126/science.1125142
- [24] A. Cavagna et al., “Scale-free correlations in the starling flocks,” Proc. Natl Acad. Sci. USA, Vol.107, No.26, pp. 11865-11870, 2010. https://doi.org/10.1073/pnas.1005766107
- [25] A. Procaccini et al., “Propagating waves in starling, Sturnus vulgaris, flocks under predation,” Anim. Behav., Vol.82, pp. 759-765, 2011. https://doi.org/10.1016/j.anbehav.2011.07.006
- [26] A. J. King et al., “Selfish-herd behaviour of sheep under threat,” Curr. Biol., Vol.22, No.14, pp. 561-562, 2012. https://doi.org/10.1016/j.cub.2012.05.008
- [27] A. Berdahl, C. J. Torney, C. C. Ioannou, J. Faria, and I. D. Couzin, “Emergent sensing of complex environments by mobile animal groups,” Science, Vol.339, No.6119, pp. 574-576, 2013. https://doi.org/10.1126/science.1225883
- [28] A. Attanasi et al., “Collective Behaviour without Collective Order in Wild Swarms of Midges,” PLoS Comput. Biol., Vol.10, Article No.e1003697, 2014. https://doi.org/10.1371/journal.pcbi.1003697
- [29] A. Strandburg-Peshkin, D. R. Farine, I. D. Couzin, and M. C. Crofoot, “Shared decision-making drives collective movement in wild baboons,” Science, Vol.348, No.6241, pp. 1358-1361, 2015. https://doi.org/10.1126/science.aaa5099
- [30] K. Tunstrøm, Y. Katz, C. C. Ioannou, C. Huepe, M. J. Lutz et al., “Collective States, Multistability and Transitional Behavior in Schooling Fish,” PLoS Comput. Biol., Vol.9, No.2, Article No.e1002915, 2013. https://doi.org/10.1371/journal.pcbi.1002915
- [31] R. Bastien and P. Romanczuk, “A model of collective behavior based purely on vision,” Science Advances, Vol.6, No.6, Article No.eaay0792, 2020. https://doi.org/10.1126/sciadv.aay0792
- [32] W. F. Bode, D. W. Franks, and A. J. Wood, “Making Noise: Emergent Stochasticity in Collective Motion,” J. Theor. Biol., Vol.267, No.3, pp. 292-299, 2010. https://doi.org/10.1016/j.jtbi.2010.08.034
- [33] W. F. Bode, D. W. Franks, and A. J. Wood, “Limited Interactions in Flocks: Relating Model Simulation to Empirical Data,” J. R. Soc. Interface, Vol.8, pp. 301-304, 2010. https://doi.org/10.1098/rsif.2010.0397
- [34] H. J. Charlesworth and M. S. Turner, “Intrinsically motivated collective motion,” Proc. Natl Acad. Sci., Vol.116, No.31, pp. 15362-15367, 2019. https://doi.org/10.1073/pnas.1822069116
- [35] P. Romanczuk, I. D. Couzin, and L. Schimansky-Geier, “Collective Motion due to Individual Escape and Pursuit Response,” Phys. Rev. Lett., Vol.102, Article No.010602, 2009. https://doi.org/10.1103/PhysRevLett.102.010602
- [36] P. Romanczuk and L. Schimansky-Geier, “Swarming and pattern formation due to selective attraction and repulsion,” Interface Focus, Vol.2, pp. 746-756, 2012. https://doi.org/10.1098/rsfs.2012.0030
- [37] J. Gautrais, F. Ginelli, R. Fournier, S. Blanco, and M. Soria, “Deciphering Interactions in Moving Animal Groups,” PLoS Comput. Biol., Vol.8, No.9, Article No.e1002678, 2012. https://doi.org/10.1371/journal.pcbi.1002678
- [38] G. Le Bon, “The crowd: A study of the popular mind,” London: Ernest Benn, 1895.
- [39] C. Feliciani, K. Shimura, and K. Nishinari, “Introduction to Crowd Management: Managing Crowds in the Digital Era: Theory and Practice,” Springer Nature, 2022.
- [40] D. Helbing and A. Johansson, “Pedestrian, crowd, and evacuation dynamics,” R. A. Meyers (Ed.), “Encyclopedia of Complexity and Systems Science,” Springer, pp. 6476-6495, 2009.
- [41] D. Helbing and P. Molnar, “Social force model for pedestrian dynamics,” Phy. Rev. E, Vol.51, pp. 4282-4286, 1995. https://doi.org/10.1103/PhysRevE.51.4282
- [42] D. Helbing, I. Farkas, and T. Vicsek, “Simulating dynamical features of escape panic,” Nature, Vol.407, pp. 487-490, 2000. https://doi.org/10.1038/35035023
- [43] C. Burstedde, K. Klauck, A. Schadschneider, and J. Zittartz, “Simulation of pedestrian dynamics using a two-dimensional cellular automaton,” Physica A, Vol.295, pp. 507-525, 2001.
- [44] A. Kirchner, H. Klüpfel, A. Schadschneider, K. Nishinari, and M. Schreckenberg, “Simulation of competitive egress behavior: Comparison with aircraft evacuation data,” Physica A, Vol.324, No.3-4, pp. 689-697, 2003. https://doi.org/10.1016/S0378-4371(03)00076-1
- [45] M. Moussaïd, N. Perozo, S. Garnier, D. Helbing, and G. Theraulaz, “The walking behaviour of pedestrian social groups and its impact on crowd dynamics,” PLoS ONE, Vol.5, Article No.e10047, 2010. https://doi.org/10.1371/journal.pone.0010047
- [46] I. Karamouzas, B. Skinner, and S. J. Guy, “Universal power law governing pedestrian interactions,” Phys. Rev. Lett., Vol.113, Article No.238701, 2014. https://doi.org/10.1103/PhysRevLett.113.238701
- [47] D. Helbing, A. Johansson, and H. Z. Al-Abideen, “Dynamics of crowd disasters: an empirical study,” Phys. Rev. E, Vol.75, Article No.046109, 2007. https://doi.org/10.1103/PhysRevE.75.046109
- [48] Y. Ma, E. W. M. Lee, M. Shi, and R. K. K. Yuen, “Spontaneous synchronization of motion in pedestrian crowds of different densities,” Nature Human Behaviour, Vol.5, No.4, pp. 447-457, 2021. https://doi.org/10.1038/s41562-020-00997-3
- [49] D. R. Parisi, A. G. Sartorio, J. R. Colonnello, A. Garcimartín, L. A. Pugnaloni, and I. Zuriguel, “Pedestrian dynamics at the running of the bulls evidence an inaccessible region in the fundamental diagram,” Proc. of the National Academy of Sciences, Vol.118, No.50, Article No.e2107827118, 2021. https://doi.org/10.1073/pnas.2107827118
- [50] M. Moussaïd, D. Helbing, and G. Theraulaz, “How simple rules determine pedestrian behavior and crowd disasters,” Proc. Natl Acad. Sci. U.S.A., Vol.108, No.17, pp. 6884-6888, 2011. https://doi.org/10.1073/pnas.1016507108
- [51] A. Johansson, “Constant-net-time headway as a key mechanism behind pedestrian flow dynamics,” Phys. Rev. E, Vol.80, Article No.026120, 2009. https://doi.org/10.1103/PhysRevE.80.026120
- [52] A. Johansson, D. Helbing, and P. K. Shukla, “Specification of the social force pedestrian model by evolutionary adjustment to video tracking data,” Adv. Complex Syst., Vol.10, pp. 271-288, 2007. https://doi.org/10.1142/S0219525907001355
- [53] A. Corbetta, J. A. Meeusen, C. M. Lee, R. Benzi, and F. Toschi, “Physics-based modeling and data representation of pairwise interactions among pedestrians,” Physical Review E, Vol.98, Article No.062310, 2018. https://doi.org/10.1103/PhysRevE.98.062310
- [54] S. Nowak and A. Schadschneider, “Quantitative analysis of pedestrian counterflow in a cellular automaton model,” Phys. Rev. E, Vol.85, Article No.066128, 2012. https://doi.org/10.1103/PhysRevE.85.066128
- [55] Q. Xu, M. Chraibi, and A. Seyfried, “Anticipation in a velocity-based model for pedestrian dynamics,” Transportation Research Part C: Emerging Technologies, Vol.133, Article No.103464, 2021. https://doi.org/10.1016/j.trc.2021.103464
- [56] Y. X. Lü, Z. X. Wu, and J. Y. Guan, “Pedestrian dynamics with mechanisms of anticipation and attraction,” Physical Review Research, Vol.2, Article No.043250, 2020. https://doi.org/10.1103/PhysRevResearch.2.043250
- [57] I. Echeverría-Huarte and A. Nicolas, “Anticipating Collisions, Navigating in Complex Environments, Elbowing, Pushing, and Smartphone-Walking: A Versatile Agent-Based Model for Pedestrian Dynamics,” arXiv Preprint, arXiv:2211.03419, 2022. https://doi.org/10.48550/arXiv.2211.03419
- [58] M. Boeckle, M. Schiestl, A. Frohnwieser, R. Gruber, R. Miller, T. Suddendorf, and N. S. Clayton, “New Caledonian crows plan for specific future tool use,” Proc. of the Royal Society B, Vol.287, Article No.20201490, 2020. https://doi.org/10.1098/rspb.2020.1490
- [59] K. Kagaya, T. Kubota, and K. Nakajima, “Self-organized criticality for dendritic readiness potential,” arXiv Preprint, arXiv:2209.09075, 2022. https://doi.org/10.48550/arXiv.2209.09075
- [60] G. Rizzolatti, L. Fogassi, and V. Gallese, “Neurophysiological mechanisms underlying the understanding and imitation of action,” Nature Reviews Neuroscience, Vol.2, pp. 661-670, 2001. https://doi.org/10.1038/35090060
- [61] E. C. Tolman, “Cognitive maps in rats and men,” Psychological Review, Vol.55, No.4, pp. 189-208, 1948. https://doi.org/10.1037/h0061626
- [62] C. Darwin, “Origin of certain instincts,” Nature, Vol.7, pp. 417-418, 1873. https://doi.org/10.1038/007417a0
- [63] G. G. Gallup Jr., “Chimpanzees: self-recognition,” Science, Vol.167, No.3914, pp. 86-87, 1970. https://doi.org/10.1126/science.167.3914.86
- [64] M. Kohda, S. Sogawa, A. L. Jordan, N. Kubo, S. Awata, S. Satoh, T. Kobayashi, A. Fujita, and R. Bshary, “Further evidence for the capacity of mirror self-recognition in cleaner fish and the significance of ecologically relevant marks,” PLoS Biology, Vol.20, Article No.e3001529, 2022. https://doi.org/10.1371/journal.pbio.3001529
- [65] K. W. Rio, G. C. Dachner, and W. H. Warren, “Local interactions underlying collective motion in human crowds,” Proc. of the Royal Society B: Biological Sciences, Vol.285, Article No.20180611, 2018. https://doi.org/10.1098/rspb.2018.0611
- [66] G. C. Dachner, T. D. Wirth, E. Richmond, and W. H. Warren, “The visual coupling between neighbours explains local interactions underlying human ‘flocking’,” Proc. of the Royal Society B, Vol.289, Article No.20212089, 2022. https://doi.org/10.1098/rspb.2021.2089
- [67] N. Tinbergen, “On aims and methods of ethology,” Zeitschrift für Tierpsychologie, Vol.20, No.4, pp. 410-433, 1963. https://doi.org/10.1111/j.1439-0310.1963.tb01161.x
- [68] R. Harpaz, A. C. Aspiras, S. Chambule, S. Tseng, M. A. Bind, F. Engert, M. C. Fishman, and A. Bahl, “Collective behavior emerges from genetically controlled simple behavioral motifs in zebrafish,” Science Advances, Vol.7, No.41, Article No.eabi7460, 2021. https://doi.org/10.1126/sciadv.abi7460
- [69] W. Tang, J. D. Davidson, G. Zhang, K. E. Conen, J. Fang, F. Serluca, J. Li, X. Xiong, M. Coble, T. Tsai, G. Molind, C. H. Fawcett, E. Sanchez, P. Zhu, I. D. Couzin, and M. C. Fishman, “Genetic Control of Collective Behavior in Zebrafish,” iScience, Vol.23, No.3, Article No.100942, 2020. https://doi.org/10.1016/j.isci.2020.100942
- [70] C. C. Ioannou, V. Guttal, and I. D. Couzin, “Predatory fish select for coordinated collective motion in virtual prey,” Science, Vol.337, No.6099, pp. 1212-1215, 2012. https://doi.org/10.1126/science.1218919
- [71] A. Berdahl, C. J. Torney, C. C. Ioannou, J. Faria, and I. D. Couzin, “Emergent sensing of complex environments by mobile animal groups,” Science, Vol.339, No.6119, pp. 574-576, 2013. https://doi.org/10.1126/science.1225883
- [72] C. Doran, D. Bierbach, J. Lukas, P. Klamser, T. Landgraf, H. Klenz, and J. Krause, “Fish waves as emergent collective antipredator behavior,” Current Biology, Vol.32, No.3, pp. 708-714, 2022. https://doi.org/10.1016/j.cub.2021.11.068
- [73] N. Mizumoto, S. Miyata, and S. C. Pratt, “Inferring collective behavior from a fossilized fish shoal,” Proc. of the Royal Society B, Vol.286, Article No.20190891, 2019. https://doi.org/10.1098/rspb.2019.0891
- [74] B. P. Burford, R. R. Williams, N. J. Demetras, N. Carey, J. Goldbogen, W. F. Gilly, and M. W. Denny, “The limits of convergence in the collective behavior of competing marine taxa,” Ecology and Evolution, Vol.12, No.3, Article No.e8747, 2022. https://doi.org/10.1002/ece3.8747
- [75] R. C. Hinz and G. G. de Polavieja, “Ontogeny of collective behavior reveals a simple attraction rule,” Proc. of the National Academy of Sciences, Vol.114, No.9, pp. 2295-2300, 2017. https://doi.org/10.1073/pnas.1616926114
- [76] C. von Krüchten and A. Schadschneider, “Empirical study on social groups in pedestrian evacuation dynamics,” Phys. A, Vol.475, pp. 129-141, 2017. https://doi.org/10.1016/j.physa.2017.02.004
- [77] S. J. Gould and E. S. Vrba, “Exaptation—a missing term in the science of form,” Paleobiology, Vol.8, No.1, pp. 4-15, 1982. https://doi.org/10.1017/S0094837300004310
- [78] H. Murakami, T. Tomaru, Y. Nishiyama, T. Moriyama, T. Niizato, and Y. P. Gunji, “Emergent runaway into an avoidance area in a swarm of soldier crabs,” PLoS One, Vol.9, Article No.e97870, 2014. https://doi.org/10.1371/journal.pone.0097870
- [79] T. Tomaru, H. Murakami, T. Niizato, Y. Nishiyama, K. Sonoda, T. Moriyama, and Y. P. Gunji, “Information transfer in a swarm of soldier crabs,” Artificial Life and Robotics, Vol.21, pp. 177-180, 2016. https://doi.org/10.1007/s10015-016-0272-y
- [80] Y. P. Gunji, H. Murakami, T. Niizato, K. Sonoda, and A. Adamatzky, “Passively Active – Actively Passive Mutual Anticipation in a Communicative Swarm,” P. L. Simeonov, L. S. Smith, and A. C. Ehresmann (Eds.), “Integral Biomathics,” pp. 169-180, Springer, Berlin, Heidelberg, 2012. https://doi.org/10.1007/978-3-642-28111-2_16
- [81] H. Murakami, T. Tomaru, T. Niizato, Y. Nishiyama, K. Sonoda, T. Moriyama, and Y. P. Gunji, “Collective behavior of soldier crab swarm in both ring-and round-shaped arenas,” Artificial Life and Robotics, Vol.20, pp. 315-319, 2015. https://doi.org/10.1007/s10015-015-0232-y
- [82] Y. P. Gunji, H. Murakami, T. Niizato, A. Adamatzky, Y. Nishiyama, M. Toda, and K. Enomoto, “An embodied swarm in co-creation,” Proc. SICE Annual Conf. 2011, pp. 2587-2589, 2011.
- [83] Y. P. Gunji, H. Murakami, T. Tomaru, and V. Basios, “Inverse Bayesian inference in swarming behaviour of soldier crabs,” Philos. Trans. R. Soc. A, Vol.376, No.2135, Article No.20170370, 2018. https://doi.org/10.1098/rsta.2017.0370
- [84] Y. P. Gunji, T. Kawai, H. Murakami, T. Tomaru, M. Minoura, and S. Shinohara, “Lévy walk in swarm models based on Bayesian and inverse Bayesian inference,” Computational and Structural Biotechnology J., Vol.19, pp. 247-260, 2021. https://doi.org/10.1016/j.csbj.2020.11.045
- [85] H. Murakami, T. Niizato, Y. Nishiyama, and Y. P. Gunji, “Inherent noise appears as a Lévy walk in fish schools,” Scientific Reports, Vol.5, No.1, Article No.10605, 2015. https://doi.org/10.1038/srep1060
- [86] T. Niizato, K. Sakamoto, Y. I. Mototake, H. Murakami, T. Tomaru, T. Hoshika, and T. Fukushima, “Finding continuity and discontinuity in fish schools via integrated information theory,” PLoS One, Vol.15, No.2, Article No.e0229573, 2020. https://doi.org/10.1371/journal.pone.0229573
- [87] T. Niizato, K. Sakamoto, Y. I. Mototake, H. Murakami, T. Tomaru, T. Hoshika, and T. Fukushima, “Four-types of IIT-induced group integrity of Plecoglossus altivelis,” Entropy, Vol.22, No.7, Article No.726, 2020. https://doi.org/10.3390/e22070726
- [88] H. Murakami, T. Niizato, and Y. P. Gunji, “A model of scale-free proportion based on mutual anticipation,” Int. J. of Artificial Life Research (IJALR), Vol.3, No.1, pp. 34-44, 2012. https://doi.org/10.4018/jalr.2012010104
- [89] H. Murakami, T. Niizato, and Y. P. Gunji, “Emergence of a coherent and cohesive swarm based on mutual anticipation,” Sci. Rep., Vol.7, Article No.46447, 2017. https://doi.org/10.1038/srep46447
- [90] P. Gerlee, K. Tunstrøm, T. Lundh, and B. Wennberg, “Impact of anticipation in dynamical systems,” Phys. Rev. E, Vol.96, No.6, Article No.062413, 2017. https://doi.org/10.1103/PhysRevE.96.062413
- [91] A. Morin, J. B. Caussin, C. Eloy, and D. Bartolo, “Collective motion with anticipation: Flocking, spinning, and swarming,” Phys. Rev. E, Vol.91, No.1, Article No.012134, 2015. https://doi.org/10.1103/PhysRevE.91.012134
- [92] D. Strömbom and A. Antia, “Anticipation Induces Polarized Collective Motion in Attraction Based Models,” Northeast J. of Complex Systems, Vol.3, No.1, Article No.2, 2021. https://doi.org/10.22191/nejcs/vol3/iss1/2
- [93] H. Charlesworth and M. Turner, “Intrinsically motivated collective motion,” Proc. of the National Academy of Sciences, Vol.116, No.31, pp. 15362-15367, 2019. https://doi.org/10.1073/pnas.1822069116
- [94] H. Murakami, C. Feliciani, and K. Nishinari, “Lévy walk process in self-organization of pedestrian crowds,” J. R. Soc. Interface, Vol.16, No.153, Article No.20180939, 2019. https://doi.org/10.1098/rsif.2018.0939
- [95] H. Murakami, Y. Nishiyama, C. Feliciani, and K. Nishinari, “Mutual anticipation can contribute to self-organization in human crowds,” Science Advances, Vol.7, No.12, Article No.eabe7758, 2021. https://doi.org/10.1126/sciadv.abe7758
- [96] H. Murakami, T. Tomaru, C. Feliciani, and Y. Nishiyama, “Spontaneous behavioral coordination between avoiding pedestrians requires mutual anticipation rather than mutual gaze,” iScience, Vol.25, No.11, Article No.105474, 2022. https://doi.org/10.1016/j.isci.2022.105474
- [97] V. Hladký and J. Havlíček, “Was Tinbergen an Aristotelian? Comparison of Tinbergen’s four whys and Aristotle’s four causes,” Human Ethology Bulletin, Vol.28, No.4, pp. 3-11, 2013.
- [98] T. Sakiyama and Y. P. Gunji, “The Müller-Lyer illusion in ant foraging,” PLoS One, Vol.8, No.12, Article No.81714, 2013. https://doi.org/10.1371/journal.pone.0081714
- [99] Y. P. Gunji, Y. Nishiyama, and A. Adamatzky, “Robust soldier crab ball gate,” Complex Systems, Vol.20, No.2, pp. 94-104, 2011. https://doi.org/10.25088/ComplexSystems.20.2.93
- [100] Y. Nishiyama, Y. P. Gunji, and A. Adamatzky, “Collision-based computing implemented by soldier crab swarms,” Int. J. of Parallel, Emergent and Distributed Systems, Vol.28, No.1, pp. 67-74, 2013. https://doi.org/10.1080/17445760.2012.662682
 This article is published under a Creative Commons Attribution-NoDerivatives 4.0 Internationa License.
This article is published under a Creative Commons Attribution-NoDerivatives 4.0 Internationa License.