Paper:
Error Evaluation Method of Approximated Inverse Kinematics for Parallel-Wire Driven System – Basic Study for Three-Wire Planar System –
Hitoshi Kino*, Nobuhiro Okubo*, Toshihide Ikeda**, and Hiroaki Ochi***
*Department of Intelligent Mechanical Engineering, Fukuoka Institute of Technology
3-30-1 Wajirohigashi, Higashi-ku, Fukuoka-shi, Fukuoka 811-0295, Japan
**Development Department, OREC Co., Ltd.
548-22 Hiyoshi, Hirokawa-machi, Yame-gun, Fukuoka 834-0195, Japan
***Department of Mechanical Engineering, Tokyo University of Science, Yamaguchi
1-1-1 Daigakudori, Sanyo-Onoda, Yamaguchi 756-0884, Japan
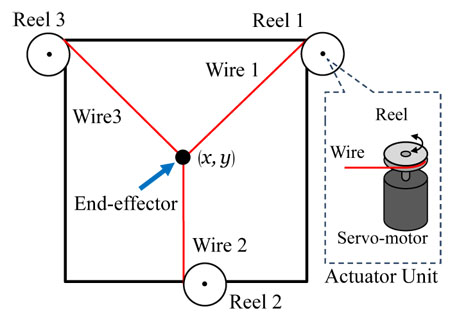
Two-degrees-of-freedom planar system using three wires
- [1] H. Kino and S. Kawamura, “Mechanism and Control of Parallel-Wire Driven System,” J. of Robotics and Mechatronics, Vol.27, No.6, pp. 599-607, 2015.
- [2] X. Tang, “An Overview of the Development for Cable-Driven Parallel Manipulator,” Advances in Mechanical Engineering, Vol.2014, pp. 1-9, 2014.
- [3] T. Bruckmann and A. Pott (Eds.), “Cable-Driven Parallel Robots (Mechanisms and Machine Science 12),” Springer-Verlag Berlin Heidelberg, 2013.
- [4] A. Pott and T. Bruckmann (Eds.), “Cable-Driven Parallel Robots: Proceedings of the Second International Conference on Cable-Driven Parallel Robots (Mechanisms and Machine Science 32),” Springer International Publishing Switzerland, 2015.
- [5] S. Kawamura, H. Kino, and W. Choe, “High speed manipulation by using parallel wire driven robots,” Robotica, Vol.18, Part 1, pp. 13-21, 2000.
- [6] H. Kino, T. Yahiro, F. Takemura, and T. Morizono, “Robust PD Control Using Adaptive Compensation For Completely Restrained Parallel-wire Driven Robots: Translational Systems Using the Minimum Number of Wires Under Zero-gravity Condition,” Trans. on Robotics, Vol.23, No.4, pp. 803-812, 2007.
- [7] H. Kino, T. Yahiro, S. Taniguchi, and K. Tahara, “Sensorless Position Control Using Feedforward Internal Force for Completely Restrained Parallel-wire Driven Systems,” Trans. on Robotics, Vol.25, No.2, pp. 467-474, 2009.
- [8] R. Clavel, “DELTA, a fast robot with parallel geometry,” Proc. Int. Symp. on Industrial Robots, pp. 91-100, 1988.
- [9] F. Pierrot, P. Dauchez, and A. Fournier, “HEXA: a fast six-DOF fully-parallel robot,” Int. Conf. on Advanced Robotics, Vol.2, pp. 1158-1163, 1991.
- [10] M. Uchiyama, “6 d.o.f. parallel robot HEXA,” Advanced Robotics, Vol.8. No.6, p. 601, 1993.
- [11] C. Jiang, K. Takagi, S. Hirano, T. Suzuki, S. Hosoe, K. Hashimoto, and A. Nozawa, “Flexible Parallel Link Mechanism Using Tube-Type Dielectric Elastomer Actuators,” J. Robotics and Mechatronics, Vol.27, No.5, pp. 504-512, 2015.
- [12] J. Albus, R. Bostelman, and N. Dagalakis, “The nist robocrane,” J. Robotic Systems, Vol.10, No.5, pp. 709-724, 1993.
- [13] H. Osumi, T. Arai, and H. Asama, “Development of a Seven Degrees of Freedom Crane with Three Wires (1st Report) – Inverse Kinematics of the Crane –,” J. Japan Society for Precision Engineering, Vol.59, No.5, pp. 767-772, 1993 (in Japanese).
- [14] A. Ming and T. Higuchi, “Study on multiple degree-of-freedom positioning mechanism using wires (part 1) – concept, design and control,” Int. J. Japan Social Engineering, Vol.28, No.2, pp. 131-138, 1994.
- [15] A. Ming and T. Higuchi, “Study on multiple degree-of-freedom positioning mechanism using wires (part 2) – development of a planar completely restrained positioning mechanism,” Int. J. Japan Social Engineering, Vol.28, No.3, pp. 235-242, 1994.
- [16] R. Lindemann and D. Tesar, “Construction and Demonstration of a 9-String 6-DOF Force Reflecting Joystick for Telerobotics,” Proc. NASA conf. Space Telerobotics, Vol.4, pp. 55-63, 1989.
- [17] Y. Hirata and M. Sato, “3-Dimensional Interface Device for Virtual Work Space,” Proc. Int. Conf. on Intelligent Robots and Systems, Vol.2, pp. 889-896, 1992.
- [18] S. Kawamura and K. Itoh, “New Type Master Robot for Teleoperation Using A Radial Wire Drive System,” Proc. of Int. Conf. on Intelligent Robots and Systems, Vol.1, pp. 55-60, 1993.
- [19] D. Yamaguchi, Y. Tagawa, M. Hayatsu, and M. Yamada, “Sequential Identification Technique of Jacobian Matrix for a Power-Assisted Lifter Using Wire-Driven Parallel Mechanism,” J. Robotics and Mechatronics, Vol.16, No.3, pp. 228-236, 2004.
- [20] P. H. Borgstrom, B. L. Jordan, B. J. Borgstrom, M. J. Stealey, G. S. Sukhatme, M. A. Batalin, and W. J. Kaiser, “NIMS-PL: A Cable-Driven Robot With Self-Calibration Capabilities” Trans. on Robotics, pp. 1005-1015, Vol.25, No.5, 2009.
- [21] P. Miermeister, A. Pott, and A. Verl, “Auto-Calibration Method for Overconstrained Cable-Driven Parallel Robots,” Proc. Int. Sympo. Robotics, pp. 1-6, 2012.
- [22] P. Miermeister, A. Pott, and A. Verl, “Dynamic Modeling and Hardware-In-The-Loop Simulation for the Cable-Driven Parallel Robot IPAnema,” Proc. Int. Sympo. Robotics, pp. 1-8, 2010.
- [23] M. C. Lei and D. Oetomo, “Cable Wrapping Phenomenon in Cable-Driven Parallel Manipulators,” J. Robotics and Mechatronics, Vol.28, No.3, pp. 386-396, 2016.
- [24] Y. Cai, S. Wang, M. Ishii, and M. Sato, “Position Measurement Improvement on a Force Display Device Using Tensed Strings,” IEICE Trans. Information and Systems Vol.E79-D, No.6, pp. 792-798, 1996.
- [25] A. Alikhani and M. Vali, “Modeling and robust control of a new large scale suspended cable-driven robot under input constraint,” Proc. Int. Conf. Ubiquitous Robots and Ambient Intelligence, pp. 238-243, 2011.
- [26] T. Dallej, M. Gouttefarde, N. Andreff, M. Micael, and P. Martinet, “Towards vision-based control of cable-driven parallel robots,” Proc. Int. Conf. Intelligent Robots and Systems, pp. 2855-2860, 2011.
- [27] E. Laroche, R. Chellal, L. Cuvillon, and J. Gangloff, “A Preliminary Study for H∞ Control of Parallel Cable-Driven Manipulators,” Cable-Driven Parallel Robots, Vol.12 of the series Mechanisms and Machine Science, pp. 353-369, Springer-Verlag Berlin Heidelberg, 2013.
 This article is published under a Creative Commons Attribution-NoDerivatives 4.0 Internationa License.
This article is published under a Creative Commons Attribution-NoDerivatives 4.0 Internationa License.