Top > JRM > On High-Performance Airfoil at Very Low Reynolds Number

single-rb.php

JRM Vol.28 No.3 pp. 273-285
doi: 10.20965/jrm.2016.p0273
(2016)

Paper:

Views over last 60 days: 2,149

On High-Performance Airfoil at Very Low Reynolds Number

Katsuya Hirata*, Ryo Nozawa*, Shogo Kondo*, Kazuki Onishi*, and Hirochika Tanigawa**

*Department of Mechanical Engineering, Doshisha University
1-3 Tatara Miyakodani, Kyotanabe, Kyoto 610-0321, Japan

**Department of Mechanical Engineering, National Institute of Technology, Maizuru College
234 Shiroya, Maizuru 625-8511, Japan

Received:
November 30, 2015
Accepted:
April 24, 2016
Published:
June 20, 2016
Creative Commons License
Keywords:
low Reynolds number, airfoil, blade, wing, aerodynamics
Abstract
The airfoil is often used as the elemental device for flying/swimming robots, determining its basic performances. However, most of the aerodynamic characteristics of the airfoil have been investigated at Reynolds numbers Re’s more than 106. On the other hand, our knowledge is not enough in low Reynolds-number ranges, in spite of the recent miniaturisation of robots. In the present study, referring to our previous findings (Hirata et al., 2011), we numerically examine three kinds of high-performance airfoils proposed for very-low Reynolds numbers; namely, an iNACA0015 (the NACA0015 placed back to front), an FPBi (a flat plate blended with iNACA0015 as its upper half) and an FPBN (a flat plate blended with the NACA0015 as its upper half), in comparison with such basic airfoils as a NACA0015 and an FP (a flat plate), at a Reynolds number Re = 1.0 × 102 using two- and three-dimensional computations. As a result, the FPBi shows the best performance among the five kinds of airfoils.
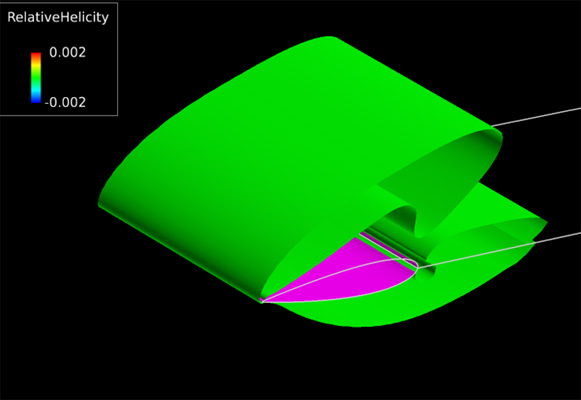
Iso-Q surfaces of very-slow flow past an iNACA0015

Iso-Q surfaces of very-slow flow past an iNACA0015

Cite this article as:
K. Hirata, R. Nozawa, S. Kondo, K. Onishi, and H. Tanigawa, “On High-Performance Airfoil at Very Low Reynolds Number,” J. Robot. Mechatron., Vol.28 No.3, pp. 273-285, 2016.
Data files:
References
  1. [1] E. N. Jacobs and A. Sherman, “Airfoil Section Characteristics as Affected by Variations in the Reynolds Number,” NACA Technical Report, No.586, pp. 227-267, 1937.
  2. [2] I. H. Abbott and A. E. von. Doenhoff, “Theory of Wing Sections,” Dover, pp. 462-463, 1958.
  3. [3] F. W. Riegels, “Aerofoil Sections,” Butterworths, 1961.
  4. [4] R. Eppler, “Airfoil Design and Data,” Springer-Verlag, 1990.
  5. [5] H. Kawano, “Three-Dimensional Obstacle Avoidance of Blimp-Type Unmanned Aerial Vehicle Flying in Unknown and Non-Uniform Wind Disturbance,” J. of Robotics and Mechatronics, Vol.19, No.2, pp. 166-173, 2007.
  6. [6] K. Iwata, K. Matsubara, K. Kawasaki, and O. Matsumoto, “Turbojet Engine for Aerial Cargo Robot (ACR),” J. of Robotics and Mechatronics, Vol.24, No.6, pp. 1040-1045, 2012.
  7. [7] J. H. McMasters and M. L. Henderson, “Low Speed Single Element Airfoil Synthesis,” Tech. Soaring, Vol.6, pp. 1-21, 1980.
  8. [8] M. Okamoto, K. Yasuda, and A. Azuma, “Aerodynamic Characteristics of the Wings and Body of a Dragonfly,” J. of Experimental Biology, Vol.199, pp. 281-294, 1996.
  9. [9] H. Abe, Y. Tutui, and H. Yoshiki, “Aerodynamic Characteristics of an Airfoil in Turbulent and Low-Reynolds-No.Flows: Experiments with a Jet Grid,” Trans. of the Japan Society of Mechanical Engineers, Series B, Vol.62, No.602, pp. 3592-3598, 1996 (in Japanese).
  10. [10] S. Sunada, A. Sakaguchi, and K. Kawachi, “Airfoil Section Characteristics at a Low Reynolds Number,” Trans. ASME, J. of Fluids Engineering, Vol.199, pp. 129-135, 1997.
  11. [11] H. Abe, “Aerodynamic Characteristics of an Airfoil in Turbulent and Low-Reynolds-No.Flows,” Technical Report of Mechanical Engineering Laboratory, AIST, Vol.186, 2000 (in Japanese).
  12. [12] H. Isikawa, D. Kudo, M. Kiya, O. Mochizuki, and Z. Zheng, “Aerodynamic Characteristics of a Flat-Plate Wing with Leading-Edge Serrations,” Trans. of the Japan Society of Mechanical Engineers, Series B, Vol.67, No.655, pp. 680-687, 2001 (in Japanese).
  13. [13] T. Motohashi, “Characteristics of Rectangular Wings at Low Reynolds Numbers,” Proc. 39th Aircraft Symposium, JSASS, Paper No.3D6, pp. 1-4, 2001 (in Japanese).
  14. [14] N. Nakane, T. Tanaka, and T. Motohashi, “Aerodynamic Characteristics of NACA0012 in a Wide Reynolds No.range,” Proc. 35th Fluid Dynamics Conf., JSASS, pp. 179-182, 2003 (in Japanese).
  15. [15] Q. Sun and I. D. Boyd, “Flat-Plate Aerodynamics at Very Low Reynolds Number,” J. of Fluid Mechanics, Vol.502, pp. 199-206, 2004.
  16. [16] R. Takagi, “Aerodynamic Characteristics of NACA4402 in Low Reynolds No.Flows,” J. of the Japan Society for Aeronautical and Space Sciences, Vol.54, No.631 pp. 367-373, 2006 (in Japanese).
  17. [17] T. Ohtake, Y. Nakae, and T. Motohashi, “Nonlinearity of the Aerodynamic Characteristics of NACA0012 Aerofoil at Low Reynolds Numbers,” J. of the Japan Society for Aeronautical and Space Sciences, Vol.55, pp. 439-445, 2007 (in Japanese).
  18. [18] H. Yagi and M. Kawahara, “Optimal Shape Determination of a Body Located in Incompressible Viscous Fluid Flow,” Computer Methods in Applied Mechanical and Engineering, Vol.196, pp. 5084-5091, 2007.
  19. [19] E. Katamine, N. Nishihashi, and H. Azegami, “Shape Optimization of Steady-State Viscous Flow Fields for Drag Minimization and Lift Maximization,” Trans. of the Japan Society of Mechanical Engineers, Series B, Vol.74, No.748, pp. 2426-2433, 2008 (in Japanese).
  20. [20] T. Ohtake and T. Motohashi, “Flow Field around NACA0012 Airfoil at Low Reynolds Numbers,” J. of the Japan Society for Aeronautical and Space Sciences, Vol.57, pp. 397-404, 2009 (in Japanene).
  21. [21] K. Taira and T. Colonius, “Three-Dimensional Flows Around Low-Aspect-Ratio Flat-Plate Wings at Low Reynolds Numbers,” J. of Fluid Mechanics, Vol.623, pp. 187-207, 2009.
  22. [22] K. Hirata, M. Kawakita, T. Iijima, M. Koga, M. Kihira, and J. Funaki, “Numerical and Experimental Study on Aerodynamic Characteristics of Basic Airfoils at Low Reynolds Numbers,” J. of Fluid Science and Technology, Vol.5, pp. 447-463, 2010.
  23. [23] Y. Zhou, Md. Mahbub Alam, H. X. Yang, H. Guo, and D. H. Wood, “Fluid Forces on a Very Low Reynolds No.Airfoil and Their Prediction,” Int. J. of Heat and Fluid Flow, Vol.32, pp. 329-339, 2011.
  24. [24] S. Ebata, T. Yasuda, H. Minagawa, Y. Miyamoto, and N. Satofuka, “A Study of Cross-Sectional Shape of Wing for Underwater Glider at Low Reynolds No.Region,” Trans. of the Japan Society of Mechanical Engineers, Series B, Vol.79, No.806, pp. 12-25, 2013 (in Japanene).

Creative Commons License  This article is published under a Creative Commons Attribution-NoDerivatives 4.0 Internationa License.

*This site is desgined based on HTML5 and CSS3 for modern browsers, e.g. Chrome, Firefox, Safari, Edge, Opera.

Last updated on Apr. 22, 2024