Paper:
Sliding Mode Control of Lateral Semi-Active Suspension of High-Speed Train
Jing He, Zhitian Liu, and Changfan Zhang†
College of Electrical and Information Engineering, Hunan University of Technology
No.88 Taishan Road, Tianyuan District, Zhuzhou, Hunan 412007, China
†Corresponding author
A control algorithm for lateral semi-active suspension based on sliding mode observer is proposed to solve lateral vibration of high-speed trains caused by railway surface excitation. Firstly, the multi-degree-of-freedom vehicle dynamics model of high-speed train is revised on the basis of variation in spring coefficient caused by long-time vibration in practical engineering; Secondly, the observer based on sliding mode variable structure is designed to obtain real-time estimation of unknown interference terms using the equivalent control principle of sliding mode variable structure, which can be further used to calculate the observed values of unknown interference terms; Finally, a control algorithm based on sliding mode observer is proposed. The algorithm inputs complex unknown interference observed values into the sliding mode controller (SMC) as feedback, thereby allowing the observed values to accurately track nonlinear unknown disturbance and weaken the vibration. Stimulation and experimental verification have proven the effectiveness and feasibility of the proposed method.
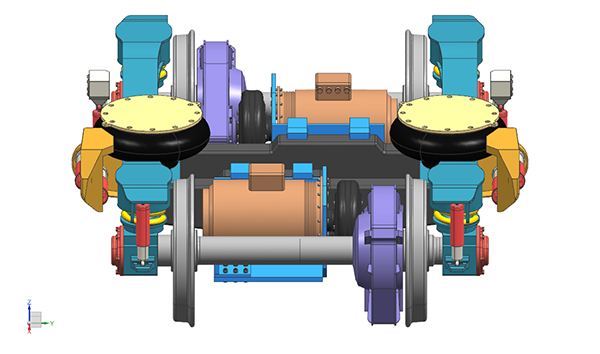
3D model of semi-active suspension system of high-speed train
- [1] W. Zhai, “Coupling dynamics of vehicle-track,” China Railway Publishing House, 2001 (in Chinese).
- [2] C. Chen and K. Wang, “Study on modeling of lateral semi-active suspension system of high-speed train,” J. of Vibration and Shock, Vol.25, No.4, pp. 151-154+169+184, 2006 (in Chinese).
- [3] S. Leva, A. P. Morando, and P. Colombaioni, “Dynamic Analysis of a High-Speed Train,” IEEE Trans. on Vehicular Technology, Vol.57, No.1, pp. 107-119, 2008.
- [4] F. Tyan and S.-H. Tu, “A Lyapunov Based Multi-level Controller for a Semi-active Suspension System with an MRF Damper,” Asian J. of Control, Vol.17, No.2, pp. 615-625, 2015.
- [5] G. R. M. Mastinu, M. Gobbi, and G. D. Pace, “Analytical formulae for the design of a railway vehicle suspension system,” Proc. of the Institution of Mechanical Engineers, Part C: J. of Mechanical Engineering Science, Vol.215, Issue 6, pp. 683-698, 2001.
- [6] D. H. Wang and W. H. Liao, “Semi-active suspension systems for railway vehicles using magnetorheological dampers. Part I: system integration and modelling,” Vehicle System Dynamics, Vol.47, Issue 11, pp. 1305-1325, 2009.
- [7] J. Marzbanrad, P. Poozesh, and M. Damroodi, “Improving vehicle ride comfort using an active and semi-active controller in a half-car model,” J. of Vibration and Control, Vol.19, Issue 9, pp. 1357-1377, 2012.
- [8] Y.-J. Shin, W.-H. You, H.-M. Hur, and J.-H. Park, “Semi-active control to reduce carbody vibration of railway vehicle by using scaled roller rig,” J. of Mechanical Science and Technology, Vol.26, Issue 11, pp. 3423-3431, 2012.
- [9] X. Wei, M. Zhu, and L. Jia, “A semi-active control suspension system for railway vehicles with magnetorheological fluid dampers,” Vehicle System Dynamics, Vol.54, Issue 7, pp. 982-1003, 2016.
- [10] J.-S. Oh et al., “Vibration control of a semi-active railway vehicle suspension with magneto-rheological dampers,” Advances in Mechanical Engineering, Vol.8, Issue 4, doi: 10.1177/1687814016643638, 2016.
- [11] Y.-J. Shin, W.-H. You, H.-M. Hur, and J.-H. Park, “H∞ control of railway vehicle suspension with MR damper using scaled roller rig,” Smart Materials and Structures, Vol.23, No.9, Article No.095023, 2014.
- [12] Q. Zhu et al., “A Low-Cost Lateral Active Suspension System of the High-Speed Train for Ride Quality Based on the Resonant Control Method,” IEEE Trans. on Industrial Electronics, Vol.65, No.5, pp. 4187-4196, 2018.
- [13] Q. Zhu, J.-J. Ding, and M.-L. Yang, “LQG control based lateral active secondary and primary suspensions of high-speed train for ride quality and hunting stability,” IET Control Theory & Applications, Vol.12, No.10, pp. 1497-1504, 2018.
- [14] J. He et al., “Virtual Line Shafting-Based Total-Amount Coordinated Control of Multi-Motor Traction Power,” J. of Advanced Transportation, Vol.2020, Article No.4735397, 2020.
- [15] J. He et al., “Demagnetization fault detection in permanent magnet synchronous motors based on sliding observer,” J. of Nonlinear Sciences and Applications, Vol.9, Issue 5, pp. 2039-2048, 2016.
- [16] D.-Y. Li, Y.-D. Song, and W.-C. Cai, “Neuro-Adaptive Fault-Tolerant Approach for Active Suspension Control of High-Speed Trains,” IEEE Trans. on Intelligent Transportation Systems, Vol.16, No.5, pp. 2446-2456, 2015.
- [17] Y. Song and X. Yuan, “Low-Cost Adaptive Fault-Tolerant Approach for Semiactive Suspension Control of High-Speed Trains,” IEEE Trans. on Industrial Electronics, Vol.63, No.11, pp. 7084-7093, 2016.
- [18] X. Yuan, Y. Song, and Z. Jia, “Adaptive PD approach for semi-active suspension control of high speed trains,” Proc. of the 2016 12th World Congress on Intelligent Control and Automation (WCICA), pp. 2672-2676, 2016.
- [19] Y.-W. Qu, C.-B. Ren, J.-L. Zhou, and B.-Z. Ma, “Semi-active suspension control by using dual-delayed feedback based on particle swarm optimization,” J. of Guangxi University (Natural Science Edition), Vol.41, No.5, pp. 1368-1376, 2016 (in Chinese).
- [20] J. Guo, Z. Xu, and Y. Sun, “A new semi-active safety control strategy for high-speed railway vehicles,” Vehicle System Dynamics, Vol.53, Issue 12, pp. 1918-1934, 2015.
- [21] J. He et al., “Robust consensus braking algorithm for distributed EMUs with uncertainties,” IET Control Theory & Applications, Vol.13, No.17, pp. 2766-2774, 2019.
- [22] J. He et al., “Integrated cooperative braking algorithm of non-linear electric multiple units with external disturbance,” The J. of Engineering, Vol.2019, Issue 23, pp. 8937-8941, 2019.
 This article is published under a Creative Commons Attribution-NoDerivatives 4.0 Internationa License.
This article is published under a Creative Commons Attribution-NoDerivatives 4.0 Internationa License.